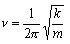
The basic topics to cover are:
(i) The vibrational energy levels and wavefunctions of a simple harmonic oscillator. The shape of the potential energy curve. The characterization of the potential energy curve in terms of dissociation energy, force constant, etc. Zero point energy. The effects of anharmonicity on the spacing of the levels.
(ii) The population of vibrational levels as a function of temperature. Contributions of vibration to the heat capacities of gases.
(iii) The vibrations of polyatomic molecules. Normal modes. Consider the vibrations of a triatomic molecule, e.g. CO2 , in detail. The use of symmetry to classify the vibrations. Selection rules for excitation of vibrational levels, including the use of group theory.
(iv) Infrared and Raman spectroscopy of polyatomic molecules. The factors that are used to assign a particular vibration to a band in the spectrum, e.g. depolarization ratios.
Almost any general book on spectroscopy will cover most of the topics above. There is an Oxford Chemistry Primer, Brown, Molecular Spectroscopy . Other suitable ones have been written by Whiffen, Banwell, Barrow, Hollis, Dixon. The books Molecular structure, and Chemical Consequences of Nuclear Spin, both by Wheatley, and very old, are useful for reading about structural determination, and bosons and fermions respectively.
Herzberg, Diatomic Molecules is more advanced but is extremely well written and is useful for reference, especially if you only read the sections in large print.
Where the question number is enclosed in a button, e.g. , you can obtain help or comments about the question by clicking it.
(i) The potential energy, V, for a harmonic oscillator is given by
V = kx2/2
where k is the force constant and x is the displacement from equilibrium. Show by direct substitution that the wave-function
ψ = Aexp(-λx2)
where
V = (mk/4h2)1/2
is a solution of the Schrodinger equation for a harmonic oscillator of mass m. Determine the corresponding energy, expressing your answer in terms of the vibrational frequency,
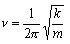
Explain why, for a diatomic molecule, the mass in the equation for the harmonic oscillator in question 1 must be replaced by the reduced mass.
Given that the stretching frequency of a C-C bond is approximately 1000
cm-1 calculate
(a) the stretching frequency of doubly and triply bound carbon atoms assuming
that the force constants are double and triple the single bond value respectively,
(b) the frequencies of a CH bond and of a Cl2 molecule on the
assumption that all single bond force constants are equal,
(c) the frequencies of the series HX where X is a halogen.
Look up the actual experimental values in each case, make a table of calculated and observed frequencies and comment on the similarities and differences between them.
(i) Use the wave function from question 1 to determine the position of maximum probability density for the harmonic oscillator wave-function in the vibrational ground state. How does the result compare (qualitatively) with the time averaged probability of finding a particle at a specific location in a classical harmonic oscillator?
The wave function for the v = 2 state is
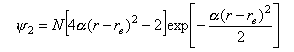
where α2 = 2π2mν2.
Plot the potential from question 1 and superimpose a plot of the square of the n = 2 wavefunction. Compare the positions of the maxima with the positions of the classical turning points for a vibration with the same amount of energy.
(ii) The figure below shows a sequence of electronic transitions (photoelectron spectroscopy) from the v = 0 state in the H2 molecule to a sequence of vibrational states in the ground state of the H2+ ion. Using the results from part (i) as a guide, explain, with the aid of a diagram, the factors responsible for the variations in peak intensity and peak separation.
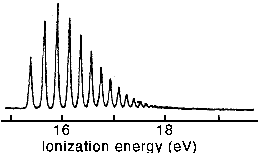
(iii) The harmonic oscillator becomes a less good approximation for vibrational energies at higher energies and, instead of being equally spaced, the levels converge. The peak positions (cm-1) in (ii) are well represented by the equation Tv = 123506 + Ev where Ev = 2322(v + 1/2) - 66.2(v + 1/2)2. Given that the spacing between successive vibrational peaks will converge to zero at the dissociation limit, estimate the dissociation energy of H2+. Why does the spacing converge to zero at the dissociation limit?
(iv) The convergence of the vibrational levels at high n can be accounted for by an anharmonic potential such as the Morse potential
V = De{1 - exp[-α(r - r e)]}2
Plot the Morse potential and superimpose a sketch of the potential for a harmonic oscillator.
The turning points of classical vibrational motion in the Morse potential at fixed energy Ev can be obtained by rearranging the above equation and are given by
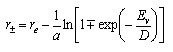
From the figure in (ii) the most probable transition is the one for which the overlap of ground and excited state wavefunctions is a maximum (n = 2 ). Use the equation above to calculate the turning points for an energy corresponding to this n = 2 state. Then, by assuming that the maximum overlap between ground and excited states occurs at the same r as the classical turning points, use your values of the turning points to calculate re for H2+. (The equilibrium bond length for H2 is re = 0.741 x 10-10 nm.)
(i) Why do atomic masses affect vibrational zero point energies of diatomic molecules? How can the zero point energy influence the rates of chemical reactions?
(ii) During the oxidation of benzaldehyde by permanganate in solution a CH(CD) bond is broken. By considering the change in zero point energies of the reactants alone, estimate the maximum ratio kH/kD of the rate constants. The vibration frequency of the CH bond is about 2900 cm-1 and you may neglect any isotopic effect on the pre-exponential term in the expression for the rate constant. What happens if the zero point energies in the transition state for the reaction cannot be neglected?
By calculating the contribution of zero point energy to the enthalpy of the reaction, calculate its effect on the ratio K(H)/K(D), at 600 K, of the equilibrium constants of the gas phase reactions

The vibration frequencies of HI and of H2 are 2300 and 4400 cm-1 respectively and the atomic weight of iodine is 127.
The frequency of the stretching vibration of CO is 2200 cm-1. In the infrared emission spectrum of the first overtone (v = 2 → 0) of CO formed in an oxy-acetylene flame a series of transitions corresponding to v = 3 → 1, 4 → 2, etc. are observed. These have slightly different frequencies because of anharmonicity (as explained in question 3). The intensities of the transitions depend on the populations of the v = 2, 3, 4, etc. levels and are proportional to
I = (v + 1)(v + 2)exp[-(v + 2)hcν/kBT]
where v refers to the lower level in each transition. Values of the intensity are
| I | 4.0 | 5.9 | 5.5 | 4.6 | 3.9 | 3.2 |
| v | 0 | 1 | 2 | 3 | 4 | 5 |
Use a graphical method to determine the temperature of the flame.
Use the following data for O2 to plot a graph of the heat capacity, CV, as a function of temperature. Discuss the shape of this graph and the origin of the temperature variation in terms of the properties of the O2 molecule. To what extent is the form of the function applicable to other molecules?
| T/K | 200 | 300 | 500 | 1000 | 1500 |
| CV/R | 2.503 | 2.533 | 2.739 | 3.197 | 3.399 |
The molecule O2 has a vibrational frequency of 1553 cm-1. Use the method of question 2 to use this frequency to estimate the vibration frequencies of F2, Cl2, Br2 and N2. Then use the heat capacity curve for O2 to estimate C V for each of the four species at 500 K.
(a) Describe the normal modes of vibration of a linear symmetrical
XY2 molecule and deduce, in terms of dipole moment
and polarisablility changes, which of the modes are infrared and/or Raman
active. How are the selection rules modified if the molecule is linear but
unsymmetric, of the type XYY or XYZ?
(b) How does the degree of
polarisation of Raman lines aid in distinguishing bond-stretching from angle
bending frequencies in the vibrational spectra of linear molecules? Interpret the observed Raman polarizations to obtain the three fundamental vibration frequencies of the linear N
2O molecule given below (all four bands are infrared active):
| Frequency/cm-1 | 2223.5 | 1285.0 | 1167.0 | 588.8 |
| Raman | polarized | polarized | polarized | absent |
In the region associated with the stretching modes of a trifluoride molecule XF3, the infrared and Raman spectra of the vapour exhibit bands with the following wavenumber (cm -1):
| Raman | Raman | Infrared | Infrared |
| 675 | strong, polarized | 675 | strong |
| 612 | weak, depolarized | 614 | very strong |
| 552 | strong, polarized | 552 | weak |
What can you deduce from these results?
For BF
3 three of the four fundamental vibrations are infrared active
and are observed at 482 (480), 718 (691) and 1505 (1454) cm-1
(the first number refers to 10BF3
and the number in brackets to 11BF
3). Assign these frequencies to three of the fundamental vibrations and identify which vibration is missing. (You may find it helpful to use the group tables to determine the nature of the normal modes
of vibration of the BF3 molecule (D
3h) and their infrared and Raman activity, but it can also been done without group theory). Other bands are observed in the infrared spectrum at the
wavenumbers shown in the table below:
| 10BF3 | 1985 | 2243 | 2385 | 3008 | 3263 |
| 11BF3 | 1932 | 2243 | 2336 | 2905 | 3214 |
These are all combinations or overtones of the four fundamentals. Using the isotopic shift and group tables to check the infrared activity of fundamentals and overtones, assign all these bands. Note that they will always be at a slightly lower frequency than expected and the accuracy is only about ± 3cm-1. Hence obtain a value of the frequency of the missing fundamental vibration.